Mrs. Napholtz's Math Site

Assorted Problems

The computer club spent $300 on a printer, the members sharing the cost equally. If there had been 5 more members in the club, each would have paid $5 less. How many members were in the club?

Hint: Cost per person = 300/(number of people).

New cost per person is 5 less than old cost per person!
300/(n + 5) = (300/n) - 5     Multiply by n(n + 5)
300n = 300(n + 5) - 5n(n + 5)
300n = 300n + 1500 - 5n² - 25n
0 = 1500 - 5n² - 25n
5n² + 25n - 1500 = 0
n² + 5n - 300 = 0
(n + 20)(n - 15) = 0
n = -20 or n = 15
Answer: 15 people

The sum of a number and its reciprocal is 3. What is (are) the number(s)?

n + (1/n) = 3      Multiply by n
n² + 1 = 3n
n² - 3n + 1 = 0      Quadratic Formula!
n = approx. 2.6 or n = approx .4

The diagonal of a square is .Find the length of a side of the square.

Again, using Pythagoras:
s² + s² = diag²
2s² = ()²
2s² = 64 * 2
s² = 64
s = 8

The velocity of a free-falling object can be found by using the formula , where g = 9.8 m/s², and d is the distance in meters. Find the distance a ball falls if its velocity is 28 m/s.

Squaring the original formula, we get:
v² = 2gd
28² = 2 * 9.8 * d
784 = 19.6d
d=40 m

Two years ago, Michael's age was 3 years less than twice Bruce's age. Four years from now, Michael's age will be 6 years more than half Bruce's age. How old is each now?

Michael will be 6 years more than half Bruce's age.
2n + 3 = 0.5(n + 6) + 6
2n + 3 = 0.5n + 3 + 6
1.5n = 6
n = 4
Two years ago, Bruce was 4 and Michael was 2n - 3 = 5
They are currently 6 and 7 years old.

The length of the hypotenuse of a right triangle is 25 units. One of the legs is 5 units longer than the other leg.

1. Set up and solve a quadratic equation to find the length of each leg.
2. Find the area of the triangle in square units.

x² + (x + 5)² = 25²
x² + x² + 10x + 25 = 625
2x² + 10x - 600 = 0
Divide by 2.     x² + 5x - 300 = 0
(x - 15)(x + 20) = 0
x = 15 OR x = -20 (not possible)
ANS: The legs are x = 15 and x + 5 = 20
Area = 1/2(15)(20) = 150 square units.

In extra help, we've learned "The Quadratic Formula" to find the solutions to ax² + bx + c = 0:

Use this formula to find the solutions to x² - 5x - 2 = 0.
Leave answers in radical form!

a = 1, b = -5, c = -2.
NOTE: b² -4ac = (-5)² -4(1)(-2) = 25 - (-8) = 33.

Simplify:

Hint: Reduce each fraction first!

Step one: Factor denominators:

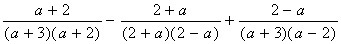
Step two: Reduce each fraction:

Step three: Note first and third terms cancel.
Step four: Multiply numerator and denominator by -1:

It is a fact that a³ - b³ = (a - b)(a² + ab + b²)
Use this fact to factor x³ - 27.
Multiply to check!

Note: For this problem, "a" = x and "b" = 3.
Using the pattern: x³ - 27 = (x - 3)(x² + x*3 + 3²)
This gives: x³ - 27 = (x - 3)(x² + 3x + 9)
Multiply to check.

It is a fact that a³ + b³ = (a + b)(a² - ab + b²)
Use this fact to factor x³ + 8.
Multiply to check!

Note: For this problem, "a" = x and "b" = 2.
Using the pattern: x³ + 8 = (x + 2)(x² - x*2 + 2²)
This gives: x³ + 8 = (x + 2)(x² - 2x + 4)
Multiply to check.

A truck delivered fertilizer that comes in 12 lb. bags and feed that comes in 15 lb. bags. If there were 6 more bags of feed than bags of fertilizer and the total delivery weighed 333 lb., find how many bags of each were delivered.

The total weight = 333
12n + 15n + 90 = 333
27n + 90 = 333
27n = 243
n = 9
Answer: 9 bags of fertilizer, 15 bags of feed.

The ratio of boys to girls in a class is 4 to 3, and there is a total of 28 students. How many girls are in the class?

Boys = 4x, Girls = 3x
4x + 3x = 28
7x = 28
x = 4
Boys: 16, Girls: 12
Answer: There are 12 girls.

Two bags of flour have a total weight of 27 pounds. If 1 pound is added to the heavier bag and 1 pound is removed from the lighter bag, the heavier bag will weigh twice as much as the lighter bag. What does the heavier bag weigh?

After the change:
(Heavier Bag) will weigh twice (The Lighter Bag)
(Heavier Bag) = 2(The Lighter Bag)
(h + 1) = 2((27 - h) - 1)    Remember: 27 - h - 1 = 26 - h
h + 1 = 2(26 - h)
h + 1 = 52 - 2h
3h + 1 = 52
3h = 51
h = 17
ANSWER: The heavier bag weighs 17 pounds.

An experienced mechanic earns $8.00 per hour more than an apprentice. If it takes the apprentice 18 hours to earn the same amount of money as the mechanic can earn in 12 hours, what is the hourly pay rate of the mechanic?

Since the two workers are earning "the same amount of money",
(Total wages of apprentice) = (Total wages of mechanic)
18a = 12a + 96
6a = 96
a = 16
a + 8 = 24
ANSWER: The hourly pay rate of the mechanic is $24.

Two years ago, Henry weighed 30 lb more than Walter. Since that time, Walter has lost 10 lb, while Henry's weight has remained the same. If the sum of their present weights is 300 lb, find how many pounds each weighs now.

Since the sum of their present weights is 300,
(Walter's present weight) + (Henry's present weight) = 300
(w - 10) + (w + 30) = 300
2w + 20 = 300
2w = 280
w = 140
w - 10 = 130
w + 30 = 170
ANSWER: Walter is now 130 pounds. Henry weighs 170 pounds.

Cindy won a game by scoring 14 points more than her father did. In a second game, Cindy's score remained the same, while her father scored twice as many points as he did in the first game. If the second game ended in a tie, find Cindy's score in the first game.

If the second game ended in a tie, then Cindy and her father had equal scores.
n + 14 = 2n
14 = n
Cindy's score in the first game was n + 14, which equals 28.

[Home]